Difference between revisions of "Elong-14-04-16"
| Line 44: | Line 44: | ||
Now, let's look at each of these a bit more indepth according to their angular momentum components | Now, let's look at each of these a bit more indepth according to their angular momentum components | ||
| − | {|class="wikitable" border="1px" align="center" cellpadding=" | + | {|class="wikitable" border="1px" align="center" cellpadding="5" cellspacing="0" style="text-align:center;" |
| − | ! | + | ! J !! <math>m_J</math> !! L !! <math>m_L</math> !! S !! <math>m_S</math> !! <math>\pi = -1^L</math> !! T !! <math>^{2S+1}L_J</math> |
| − | |||
| − | |||
|- | |- | ||
| − | | <math>| | + | | 0 || 0 || 0 || 0 || 1 || 0 || + || 1 || <math>^1 S_0</math> |
| + | |- | ||
| + | ! 1 || ±1 !! 0 || 0 !! 1 || ±1 !! + !! 0 !! <math>^3 S_1</math> | ||
| + | |- | ||
| + | | 1 || 1 || 2 || 1 || 1 || 0 || - || 0 || <math>^1 P_1</math> | ||
| + | |- | ||
| + | | 1 || 0 || 2 || -1 || 1 || 1 || - || 1 || <math>^3 P_0</math> | ||
| + | |- | ||
| + | | 1 || 0 || 2 || 1 || 1 || -1 || - || 1 || <math>^3 P_0</math> | ||
| + | |- | ||
| + | | 1 || 1 || 2 || 1 || 1 || 0 || - || 1 || <math>^3 P_1</math> | ||
| + | |- | ||
| + | | 1 || 2 || 2 || 1 || 1 || 1 || - || 1 || <math>^3 P_2</math> | ||
| + | |- | ||
| + | | 1 || 2 || 2 || 2 || 1 || 0 || + || 1 || <math>^1 D_2</math> | ||
| + | |- | ||
| + | ! 1 !! 1 !! 2 !! 2 !! 1 || -1 !! + !! 0 !! <math>^3 D_1</math> | ||
| + | |- | ||
| + | ! 1 !! -1 !! 2 !! -2 !! 1 || +1 !! + !! 0 !! <math>^3 D_1</math> | ||
| + | |- | ||
| + | | 3 || 3 || 2 || 2 || 1 || 1 || + || 0 || <math>^3 D_2</math> | ||
| + | |- | ||
| + | | 3 || 3 || 2 || 2 || 1 || 1 || + || 0 || <math>^3 D_3</math> | ||
| + | |- | ||
| + | |} | ||
| + | |||
| + | When we remove all of the states that can't exist, we're left with two: | ||
| + | |||
| + | {|class="wikitable" border="1px" align="center" cellpadding="5" cellspacing="0" style="text-align:center;" | ||
| + | ! J !! <math>m_J</math> !! L !! <math>m_L</math> !! S !! <math>m_S</math> !! <math>\pi = -1^L</math> !! T !! <math>^{2S+1}L_J</math> | ||
| + | |- | ||
| + | ! 1 || ±1 || 0 || 0 || 1 || ±1 || + || 0 || <math>^3 S_1</math> | ||
| + | |- | ||
| + | ! 1 || 1 || 2 || 2 || 1 || -1 || + || 0 || <math>^3 D_1</math> | ||
| + | |- | ||
| + | ! 1 || -1 || 2 || -2 || 1 || +1 || + || 0 || <math>^3 D_1</math> | ||
|- | |- | ||
|} | |} | ||
Revision as of 11:48, 24 April 2014
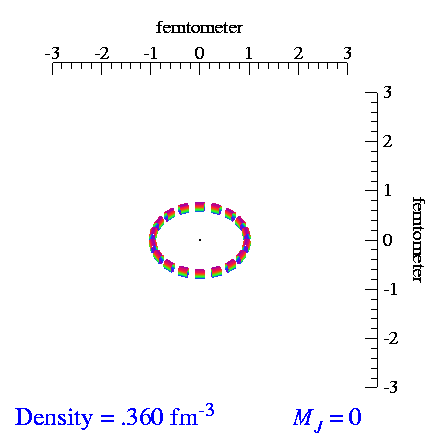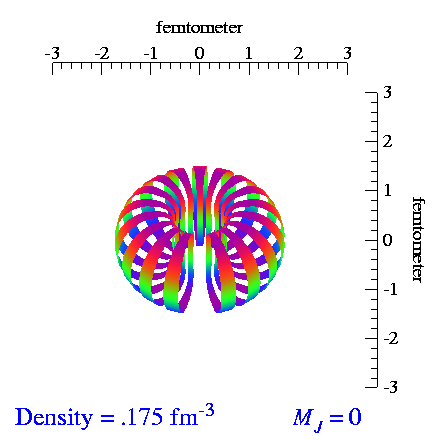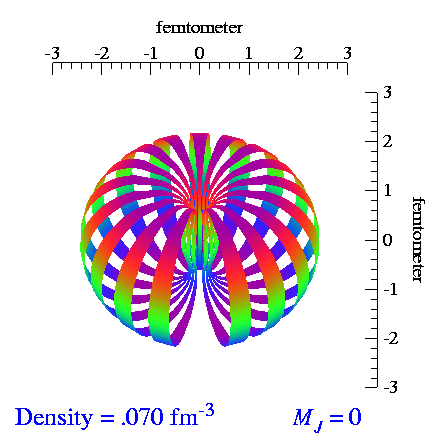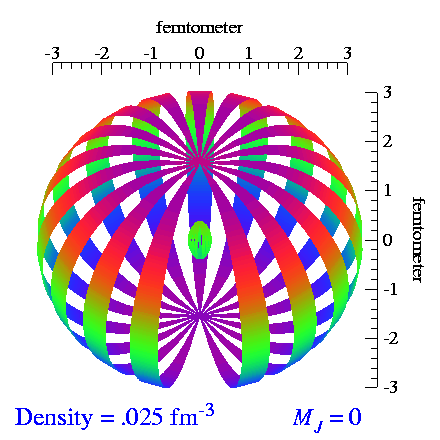
Deuteron Shape
From the video make by S.C. Pieper, et al., I extracted the tensor and vector polarization frames and made repeating videos of each. When we vector-polarize or tensor-polarize, the probability densities for the deuteron look like:
| Vector | Tensor |
|---|---|
 |
|
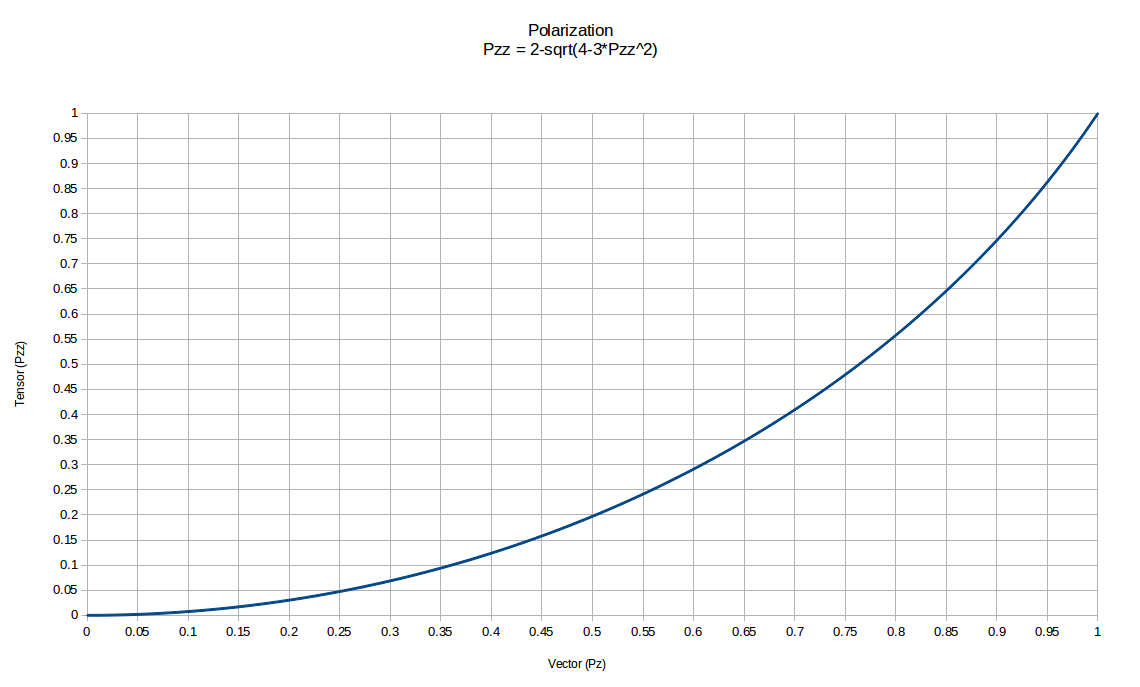
Tensor Polarization Relation to Vector Polarization
Tensor polarization is related to the vector polarization by <math>P_z=2-\sqrt{4-3P_{zz}^2}</math>
Deuteron States
From basic quantum mechanics, we know that the possible states for 2 nucleons are
the isospin singlet with S=0:
- <math>|\uparrow \downarrow> - |\downarrow \uparrow></math> with <math>m_s=0</math>, <math>L=1</math>
and the isospin triplet with S=1:
- <math>|\uparrow \uparrow></math> with <math>m_s=+1</math>, <math>L=0</math>
- <math>|\uparrow \downarrow> + |\downarrow \uparrow></math> with <math>m_s=0</math>, <math>L=1</math>
- <math>|\downarrow \downarrow></math> with <math>m_s=-1</math>, <math>L=2</math>
For the deuteron, <math>J=1</math> and <math>P=+1</math>. This kills both of the <math>m_s=0</math> states, since they cannot simultaneously have <math>J=1</math> and <math>P=+1</math> since <math>P=(-1)^L</math>.
This leaves only two possible states:
- <math>|\uparrow \uparrow></math> with <math>m_s=+1</math>, <math>L=0</math>
- <math>|\downarrow \downarrow></math> with <math>m_s=-1</math>, <math>L=2</math>
Angular Momentum Analysis
Now, let's look at each of these a bit more indepth according to their angular momentum components
| J | <math>m_J</math> | L | <math>m_L</math> | S | <math>m_S</math> | <math>\pi = -1^L</math> | T | <math>^{2S+1}L_J</math> |
|---|---|---|---|---|---|---|---|---|
| 0 | 0 | 0 | 0 | 1 | 0 | + | 1 | <math>^1 S_0</math> |
| 1 | ±1 | 0 | 0 | 1 | ±1 | + | 0 | <math>^3 S_1</math> |
| 1 | 1 | 2 | 1 | 1 | 0 | - | 0 | <math>^1 P_1</math> |
| 1 | 0 | 2 | -1 | 1 | 1 | - | 1 | <math>^3 P_0</math> |
| 1 | 0 | 2 | 1 | 1 | -1 | - | 1 | <math>^3 P_0</math> |
| 1 | 1 | 2 | 1 | 1 | 0 | - | 1 | <math>^3 P_1</math> |
| 1 | 2 | 2 | 1 | 1 | 1 | - | 1 | <math>^3 P_2</math> |
| 1 | 2 | 2 | 2 | 1 | 0 | + | 1 | <math>^1 D_2</math> |
| 1 | 1 | 2 | 2 | 1 | -1 | + | 0 | <math>^3 D_1</math> |
| 1 | -1 | 2 | -2 | 1 | +1 | + | 0 | <math>^3 D_1</math> |
| 3 | 3 | 2 | 2 | 1 | 1 | + | 0 | <math>^3 D_2</math> |
| 3 | 3 | 2 | 2 | 1 | 1 | + | 0 | <math>^3 D_3</math> |
When we remove all of the states that can't exist, we're left with two:
| J | <math>m_J</math> | L | <math>m_L</math> | S | <math>m_S</math> | <math>\pi = -1^L</math> | T | <math>^{2S+1}L_J</math> |
|---|---|---|---|---|---|---|---|---|
| 1 | ±1 | 0 | 0 | 1 | ±1 | + | 0 | <math>^3 S_1</math> |
| 1 | 1 | 2 | 2 | 1 | -1 | + | 0 | <math>^3 D_1</math> |
| 1 | -1 | 2 | -2 | 1 | +1 | + | 0 | <math>^3 D_1</math> |