Difference between revisions of "Elong-13-05-01-Azz-Method-2"
| Line 154: | Line 154: | ||
==Comparing F1 from PDFs and Bosted== | ==Comparing F1 from PDFs and Bosted== | ||
| + | '''Note: For the plots below, Phys Rate = D2 Rate Only''' | ||
| + | |||
The plot below uses F1 calculated per nucleon from the PDF code, not from the Bosted code. | The plot below uses F1 calculated per nucleon from the PDF code, not from the Bosted code. | ||
Revision as of 21:05, 2 May 2013
Azz Formalism - Method 2
Target Material = ND3 <math>z_{\mathrm{tgt}} = 3\mathrm{ cm}</math> <math>p_f = 0.65</math> <math>P_{zz} = 20\%</math> <math>N_{A} = 6.0221413\cdot 10^{23}</math> <math>\rho_{\mathrm{He}} = 0.1412 \mathrm{g/cm}^3</math> <math>M_{\mathrm{He}} = 4.0026 \mathrm{g/mole}</math> <math>\rho_{\mathrm{ND}_3} = 1.007 \mathrm{g/cm}^3</math> <math>M_{\mathrm{ND}_3} = 20 \mathrm{g/mole}</math> <math>I_{\mathrm{beam}} = 0.115 \mathrm{\mu A}</math> <math>\delta F_1^d = 5\%</math>
| (1) | <math>R_{\mathrm{Total}} = \mathcal{A}\left[ \mathcal{L}_{\mathrm{He}}\left( \frac{d^2\sigma_{\mathrm{He}}^u}{d\Omega dE'}\right) + \mathcal{L}_{\mathrm{N}}\left( \frac{d^2\sigma_{\mathrm{N}}^u}{d\Omega dE'}\right) + \mathcal{L}_{\mathrm{D}}\left( \frac{d^2\sigma_{\mathrm{D}}}{d\Omega dE'}\right) \right]</math> |
| (2) | <math>R_{\mathrm{Total}} = \mathcal{A}\left[ \mathcal{L}_{\mathrm{He}}\left( \frac{d^2\sigma_{\mathrm{He}}^u}{d\Omega dE'}\right) + \mathcal{L}_{\mathrm{N}}\left( \frac{d^2\sigma_{\mathrm{N}}^u}{d\Omega dE'}\right) + \mathcal{L}_{\mathrm{D}}\left( \frac{d^2\sigma_{\mathrm{D}}^u}{d\Omega dE'}\left[ 1 + \frac{1}{2}P_{zz}A_{zz}^d \right]\right) \right] </math> |
| (2a) | <math>R_{\mathrm{Total}} = \mathcal{A}\left[ \mathcal{L}_{\mathrm{He}} \sigma_{\mathrm{He}}^u + \mathcal{L}_{\mathrm{N}} \sigma_{\mathrm{N}}^u + \mathcal{L}_{\mathrm{D}} \sigma_{\mathrm{D}}^u\left( 1 + \frac{1}{2}P_{zz}A_{zz}^d \right) \right] </math> |
where
| (3) | <math>\mathcal{A} = \left( \Delta\Omega \Delta E' \right)</math> |
| (4) | <math>\mathcal{L}_{\mathrm{He}} = \left[ \mathcal{N}_A \frac{\rho_{\mathrm{He}}}{M_{\mathrm{He}}}\left(1 - p_f\right) \right] \cdot \left( \frac{I_{\mathrm{beam}}}{e} \right) \cdot z_{\mathrm{tgt}}</math> |
| (5) | <math>\mathcal{L}_{\mathrm{N}} = \left[ \mathcal{N}_A \frac{\rho_{\mathrm{ND}_3}}{M_{\mathrm{ND}_3}} p_f \right] \cdot \left( \frac{I_{\mathrm{beam}}}{e} \right) \cdot z_{\mathrm{tgt}}</math> |
| (6) | <math>\mathcal{L}_{\mathrm{D}} = 3\left[ \mathcal{N}_A \frac{\rho_{\mathrm{ND}_3}}{M_{\mathrm{ND}_3}} p_f \right] \cdot \left( \frac{I_{\mathrm{beam}}}{e} \right) \cdot z_{\mathrm{tgt}}</math> |
| (7) | <math>\sigma^u_X = \frac{d^2\sigma^u_X}{d\Omega dE'} = A_X \left( \frac{d\sigma}{d\Omega} \right) _{\mathrm{Mott}_{\mathrm{p}}} \left[ \frac{2\cdot \left(\frac{F_1^{X}}{A_X} \right)}{m_{p}}\tan^2\left( \frac{\theta_{e'}}{2} \right) + \frac{\left( \frac{F_2^X}{A_X}\right) }{\nu} \right]</math> |
| (8) | <math>\left( \frac{d\sigma}{d\Omega} \right) _{\mathrm{Mott}_{\mathrm{p}}}=\frac{1^2 \alpha^2 \hbar^2 c^2}{4E^2\sin^4\left( \frac{\theta}{2} \right)}\cos^2\left( \frac{\theta}{2} \right)</math> |
| (9) | <math>\sigma_D = \frac{d^2\sigma_{\mathrm{D}}}{d\Omega dE'} = \frac{d^2\sigma_{\mathrm{D}}^u}{d\Omega dE'}\left[ 1 + \frac{1}{2}P_{zz}A_{zz}^d \right] </math> |
Then
| (10) | <math>N_{Pol} - N_{u} = R^{Pol}_{\mathrm{Total}}t^{Pol}_{\mathrm{Total}} - R^{u}_{\mathrm{Total}}t^{u}_{\mathrm{Total}} </math> |
| (11) | <math>N_{u} = R^{u}_{\mathrm{Total}}t^{u}_{\mathrm{Total}} </math> |
If we assume that <math>t^{Pol}_{\mathrm{Total}} \approx t^{u}_{\mathrm{Total}} \approx t</math>, then
| (12) | <math>N_{Pol} - N_{u} = \left( R^{Pol}_{\mathrm{Total}} - R^{u}_{\mathrm{Total}}\right) t </math> |
| (13) | <math>N_{Pol} - N_{u} = \left( \mathcal{A}\left[ \mathcal{L}_{\mathrm{He}} \sigma_{\mathrm{He}}^u + \mathcal{L}_{\mathrm{N}} \sigma_{\mathrm{N}}^u + \mathcal{L}_{\mathrm{D}} \sigma_{\mathrm{D}}^u\left( 1 + \frac{1}{2}P_{zz} A_{zz}^d \right) \right] - \mathcal{A}\left[ \mathcal{L}_{\mathrm{He}} \sigma_{\mathrm{He}}^u + \mathcal{L}_{\mathrm{N}} \sigma_{\mathrm{N}}^u + \mathcal{L}_{\mathrm{D}} \sigma_{\mathrm{D}}^u \right] \right) t </math> |
| (13a) | <math>N_{Pol} - N_{u} = \left( \mathcal{A}\mathcal{L}_{\mathrm{D}}\sigma_{\mathrm{D}}^u \left( \frac{1}{2}P_{zz} A_{zz} \right) \right) t</math> |
| (14) | <math>N_{u} = R^{u}_{\mathrm{Total}}t </math> |
| (15) | <math>N_{u} = \mathcal{A}\left[ \mathcal{L}_{\mathrm{He}} \sigma_{\mathrm{He}}^u + \mathcal{L}_{\mathrm{N}} \sigma_{\mathrm{N}}^u + \mathcal{L}_{\mathrm{D}} \sigma_{\mathrm{D}}^u \right]t </math> |
| (16) | <math>\frac{N_{Pol} - N_{u}}{N_{u}} = \left( \frac{\mathcal{L}_{\mathrm{D}}\sigma_{\mathrm{D}}^u}{ \mathcal{L}_{\mathrm{He}} \sigma_{\mathrm{He}}^u + \mathcal{L}_{\mathrm{N}} \sigma_{\mathrm{N}}^u + \mathcal{L}_{\mathrm{D}}\sigma_{\mathrm{D}}^u } \right) \frac{1}{2} A_{zz} P_{zz}</math> |
| (17) | <math>\frac{N_{Pol} - N_{u}}{N_{u}} = f \frac{1}{2} A_{zz} P_{zz}</math> |
| (18) | <math>A_{\mathrm{meas}}^{(2)} = \frac{N_{Pol} - N_{u}}{N_{u}}</math> |
| (18a) | <math>A_{\mathrm{meas}}^{(2)} = f \frac{1}{2} A_{zz} P_{zz}</math> |
| (19) | <math>A_{zz} = \frac{2 }{f \cdot P_{zz}}A_{\mathrm{meas}}^{(2)}</math> |
In order to get the uncertainty, we'd use
| (20) | <math>\delta A_{zz} = \sqrt{\left( \frac{\partial A_{zz}}{\partial A_{\mathrm{meas}}^{(2)}} \delta A_{\mathrm{meas}}^{(2)} \right)^2 + \left( \frac{\partial A_{zz}}{\partial f} \delta f \right)^2 + \left( \frac{\partial A_{zz}}{\partial P_{zz}} \delta P_{zz} \right)^2 }</math> |
| (20a) | <math>\delta A_{zz} = \sqrt{\left( \delta A_{zz}^{\mathrm{Stat}} \right) ^2 + \left( \delta A_{zz}^{\mathrm{Dil}} \right) ^2 + \left( \delta A_{zz}^{\mathrm{Pol}} \right) ^2 }</math> |
| (20b) | <math>\delta A_{zz} = \sqrt{\left( \delta A_{zz}^{\mathrm{Stat}} \right) ^2 + \left( \delta A_{zz}^{\mathrm{Sys}} \right) ^2 }</math> |
Ignoring <math>\delta A_{zz}^{\mathrm{Sys}}</math> for now (and in all of the plots I'm showing), then
| (21) | <math> \delta A_{zz}^{\mathrm{Stat}} = \frac{\partial A_{zz}}{\partial A_{\mathrm{meas}}^{(2)}} \delta A_{\mathrm{meas}}^{(2)} = \frac{2}{f\cdot P_{zz}} \delta A_{\mathrm{meas}}^{(2)} </math> |
| (22) | <math> \delta A_{\mathrm{meas}}^{(2)} = \sqrt{ \left( \frac{\partial A_{\mathrm{meas}}^{(2)}}{\partial N_{Pol}} \delta N_{Pol} \right)^2 + \left( \frac{\partial A_{\mathrm{meas}}^{(2)}}{\partial N_{u}} \delta N_{u} \right)^2 }</math> |
| (23) | <math> \delta A_{\mathrm{meas}}^{(2)} = \sqrt{ \left( \frac{1}{N_u} \sqrt{N_{Pol}} \right)^2 + \left( -\frac{N_{Pol}}{N_u^2} \sqrt{N_u} \right)^2 }</math> |
| (24) | <math> \delta A_{\mathrm{meas}}^{(2)} = \sqrt{ \frac{N_{Pol}}{N_u^2} + \frac{N_{Pol}^2}{N_u^3} }</math> |
If we assume that <math>N_{Pol} \approx N_u \approx \frac{N}{2}</math>, then
| (25) | <math> \delta A_{\mathrm{meas}}^{(2)} = \sqrt{ \frac{N/2}{N^2/4} + \frac{N^2/4}{N^3/8} }</math> |
| (25a) | <math> \delta A_{\mathrm{meas}}^{(2)} = \sqrt{ \frac{2}{N} + \frac{2}{N} }</math> |
| (25b) | <math> \delta A_{\mathrm{meas}}^{(2)} = \frac{2}{\sqrt{N}}</math> |
which would yield
| (26) | <math> \delta A_{zz}^{\mathrm{Stat}} = \frac{2}{f\cdot P_{zz}} \delta A_{\mathrm{meas}}^{(2)} </math> |
| (26a) | <math> \delta A_{zz}^{\mathrm{Stat}} = \frac{2}{f\cdot P_{zz}} \left( \frac{2}{\sqrt{N}} \right) </math> |
| (27) | <math> \delta A_{zz}^{\mathrm{Stat}} = \frac{4}{f\cdot P_{zz}\sqrt{t\cdot R_{\mathrm{Total}}}} </math> |
Using the same formalism that HERMES used (which defines <math>F_{1_{\mathrm{HERMES}}}^d = \frac{(1 + Q^2/\nu^2)F_2^d}{2x(1+R)}</math> with <math>F_2^d=\frac{F_2^p + F_2^n}{2}</math> as a per nucleon quantity, which corresponds to the Bosted that uses per nucleus by <math>F_{1_{\mathrm{HERMES}}}^d = \frac{F_{1_{\mathrm{Bosted}}}^d}{A_{\mathrm{D}}} = \frac{F_1^d}{2}</math> -- as described previously), we can extract <math>b_1^d</math> and its uncertainty by
| (18) | <math> b_1^d = - \frac{3}{2}A_{zz} \left( \frac{F_1^d}{A_{\mathrm{D}}} \right)= - \frac{3}{2}A_{zz} \left( \frac{F_1^d}{2} \right)</math> |
| (19) | <math> \delta b_1^d =\sqrt{ \left(\frac{\partial b_1^d}{\partial A_{zz}} \delta A_{zz} \right)^2 + \left(\frac{\partial b_1^d}{\partial F_1^d} \delta F_1^d \right)^2 }</math> |
| (19a) | <math> \delta b_1^d =\sqrt{ \left[ - \frac{3}{2} \left( \frac{F_1^d}{2} \right)\delta A_{zz} \right]^2 + \left[ - \frac{3}{2} A_{zz} \left( \frac{1}{2} \right)\delta F_1^d \right]^2 }</math> |
Plots with using Azz Method 2 Calculations
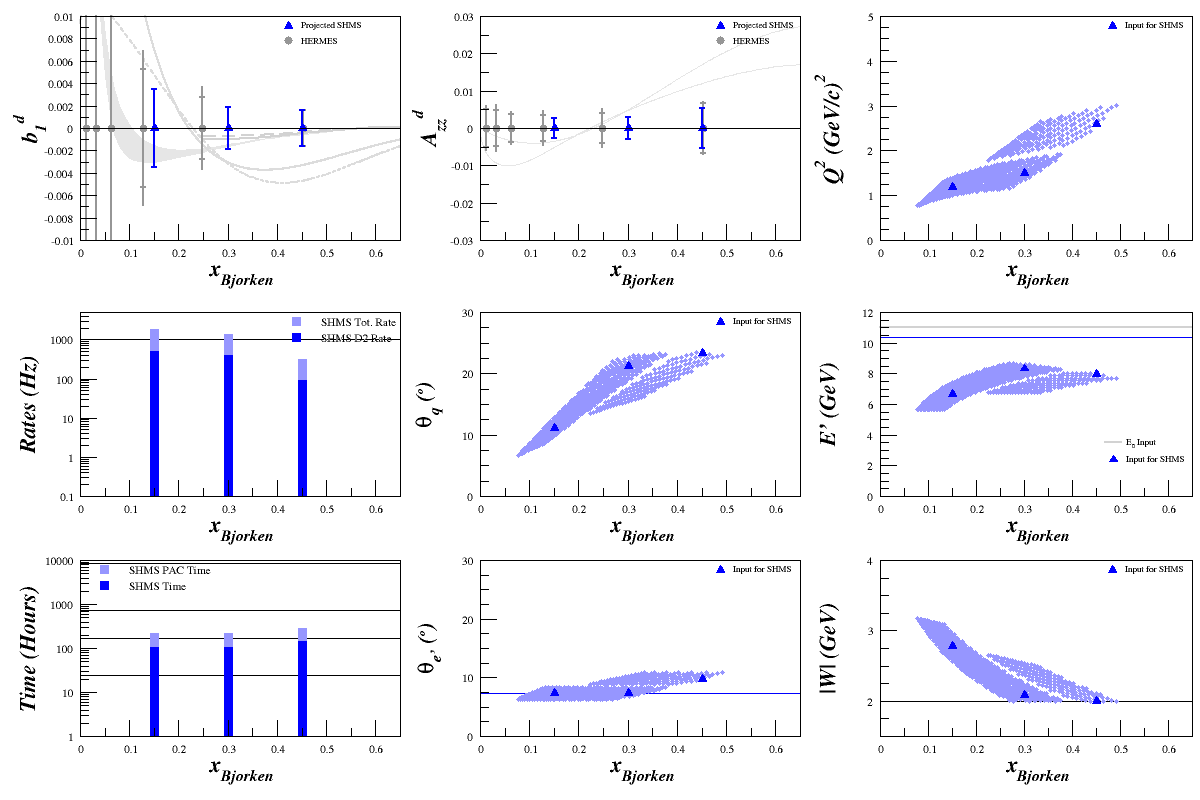
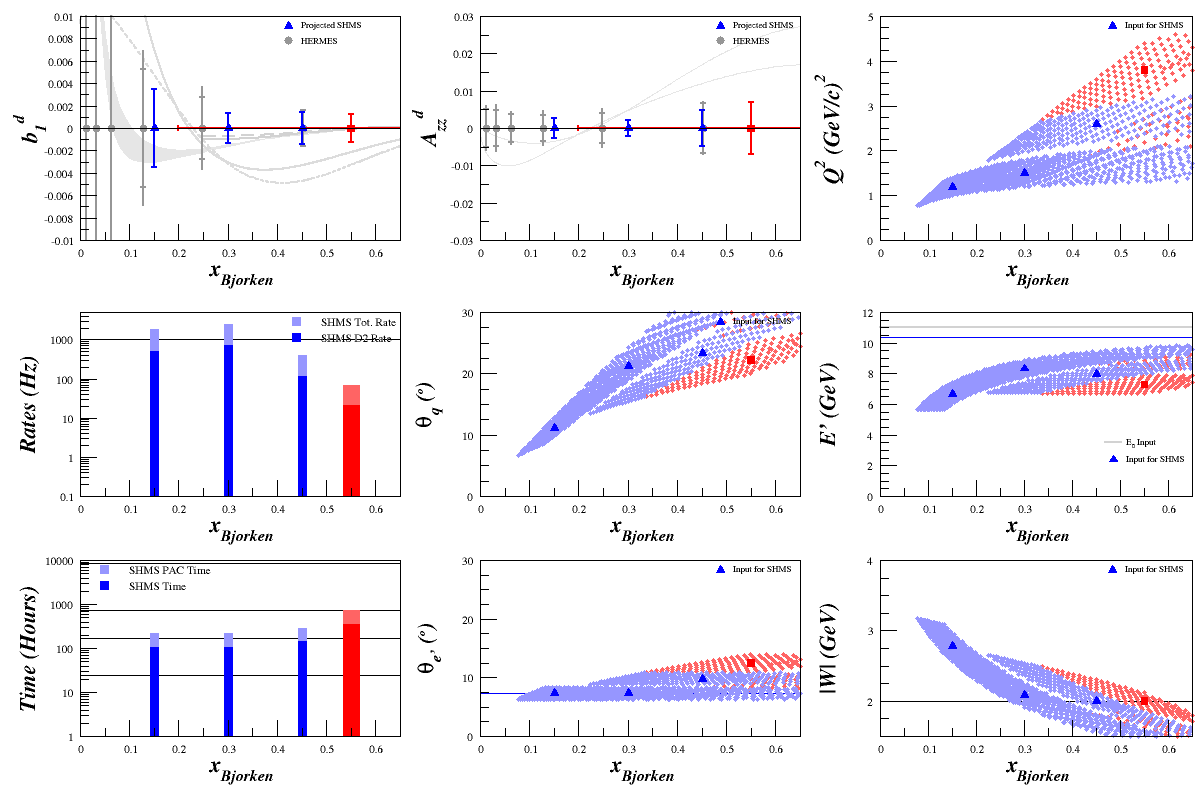
Total Rates
Ignoring our W>2 physics cut, then our total rates come out to:
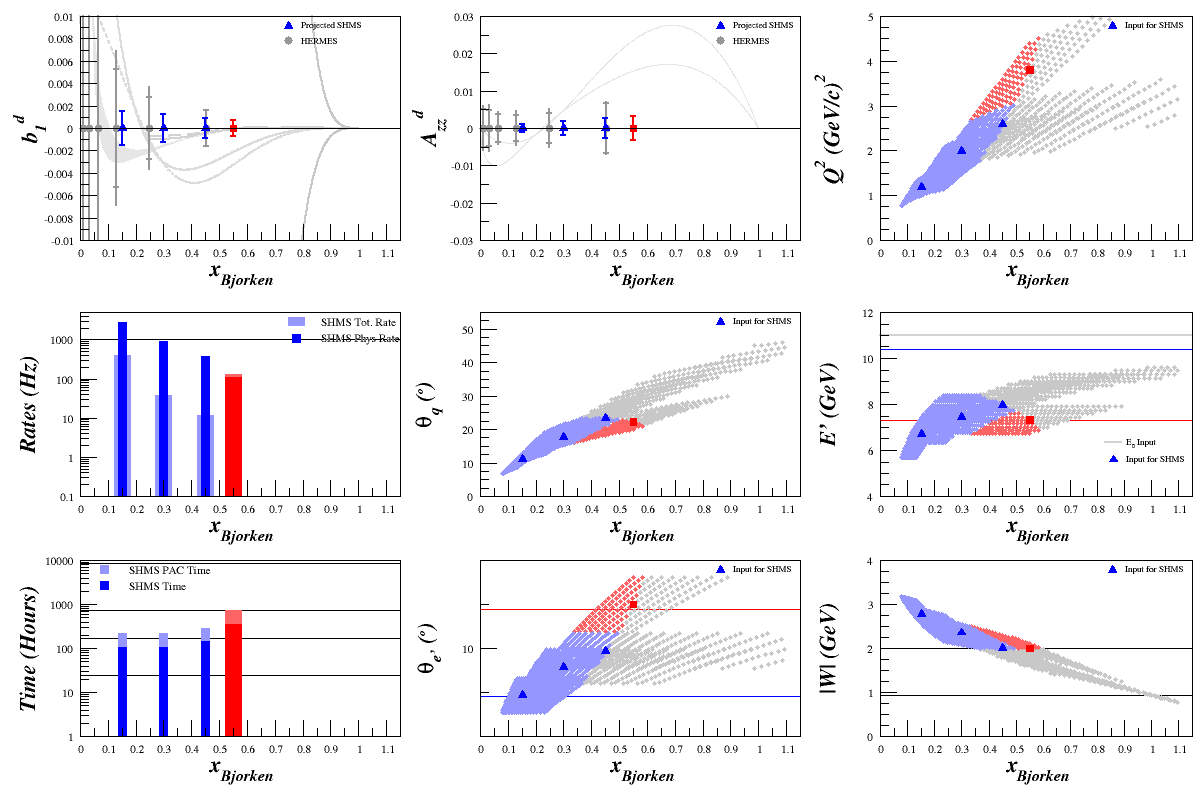
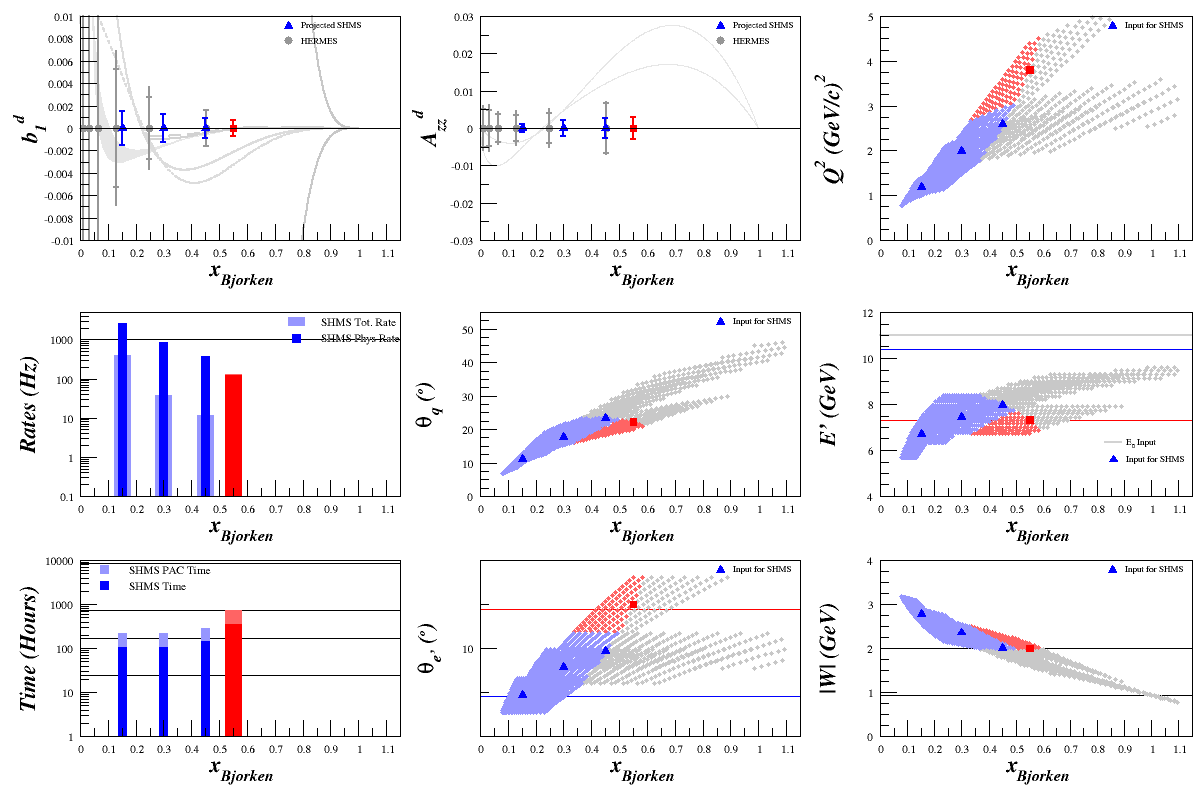
Comparing F1 from PDFs and Bosted
Note: For the plots below, Phys Rate = D2 Rate Only
The plot below uses F1 calculated per nucleon from the PDF code, not from the Bosted code.
If I calculate F1 per nucleon from the Bosted code, I get: