Difference between revisions of "Elong-14-04-15"
| Line 25: | Line 25: | ||
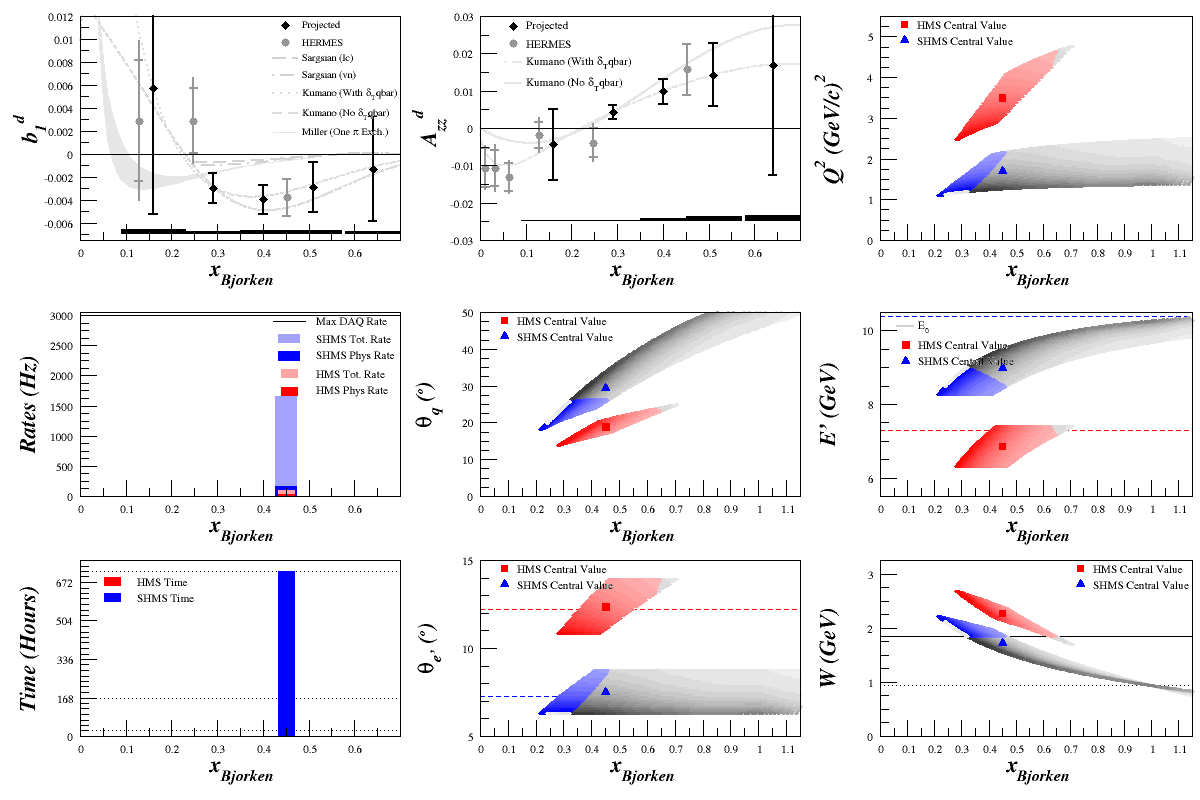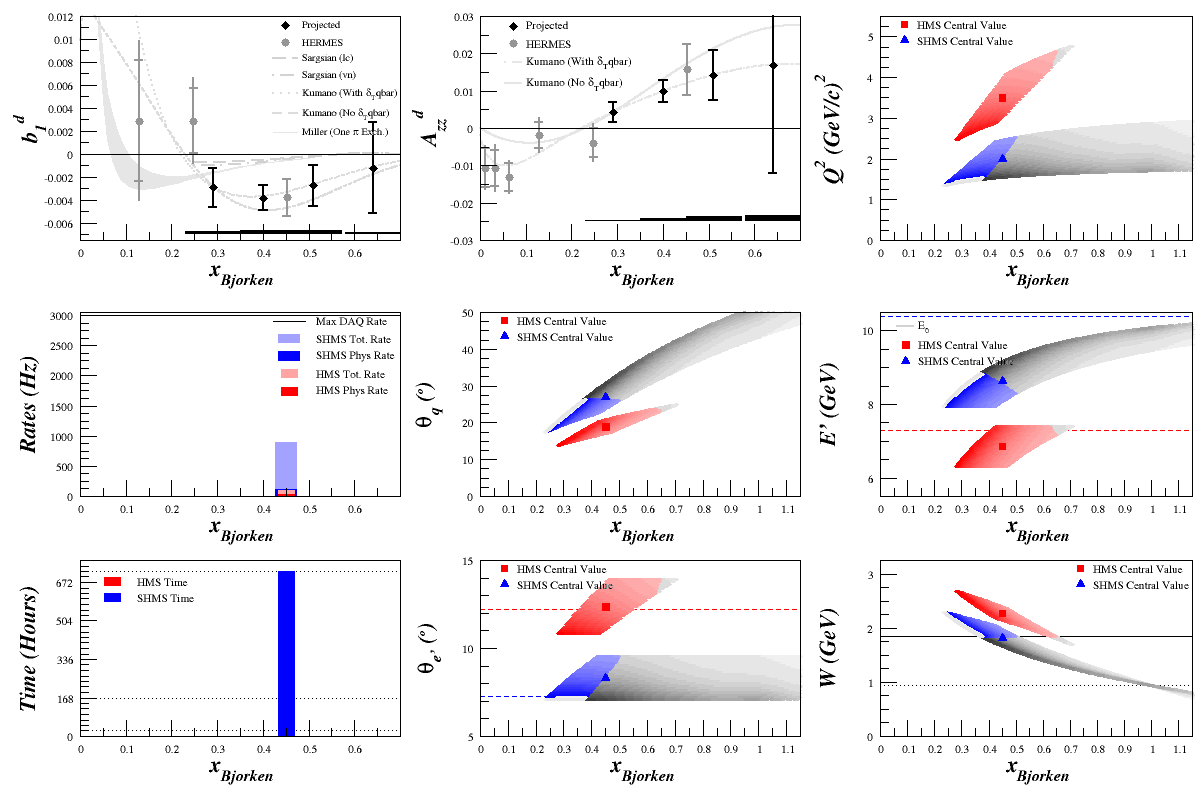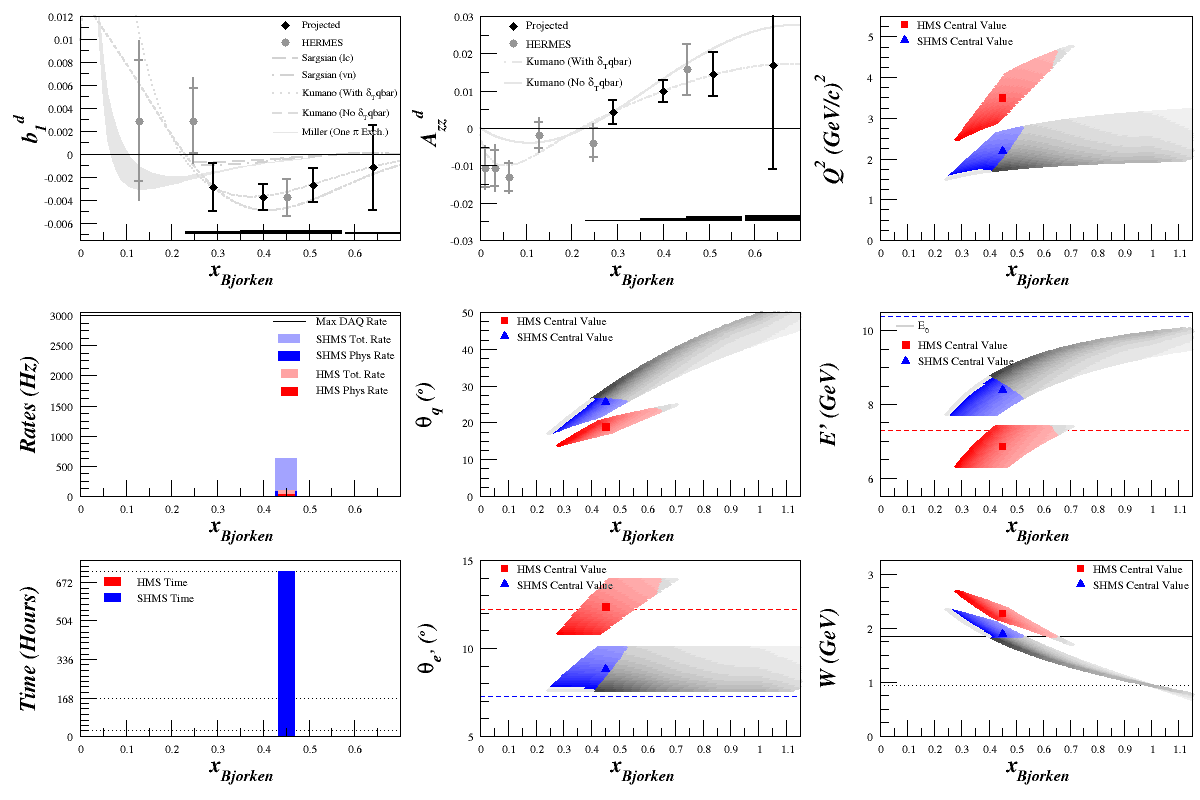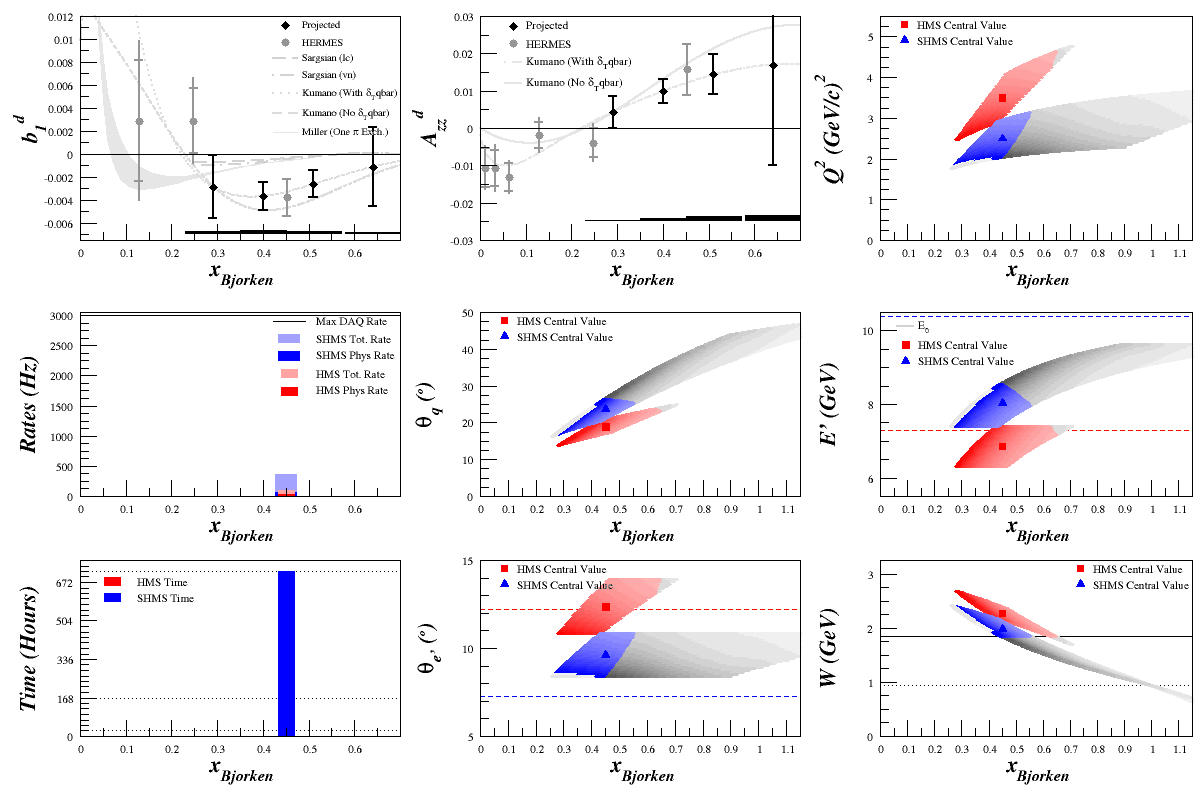
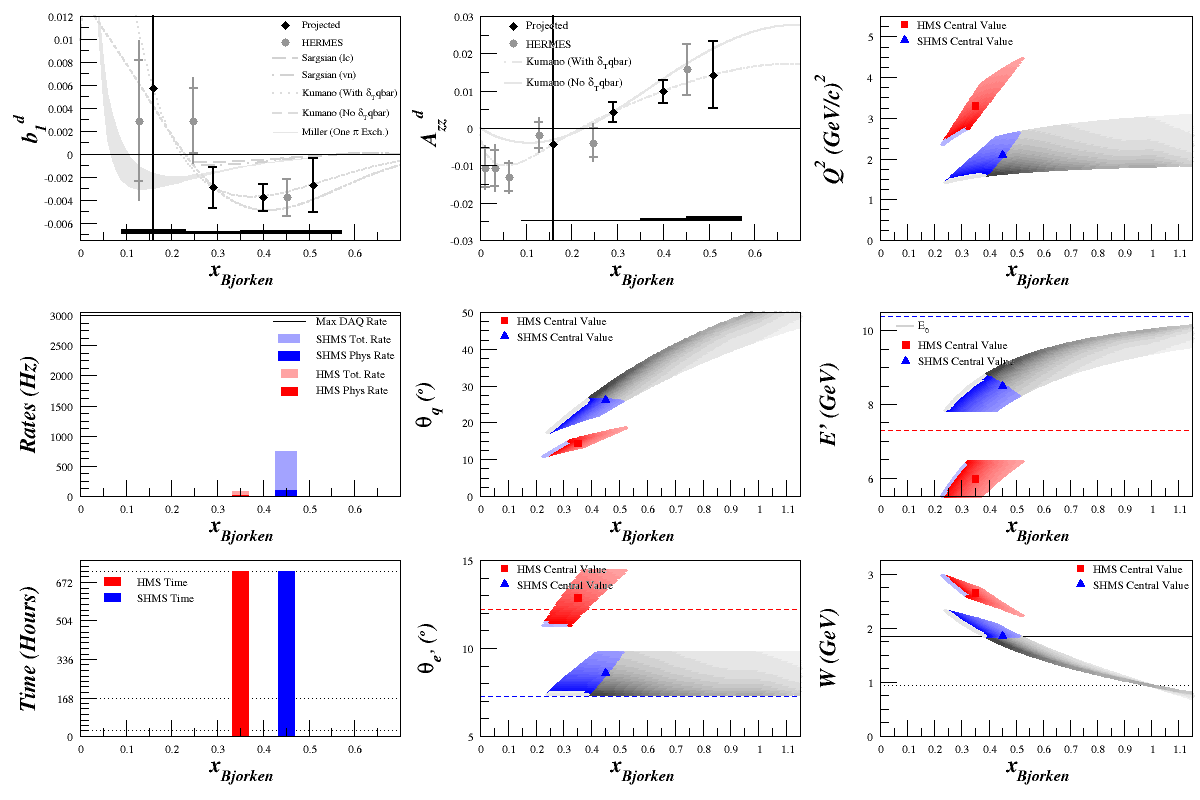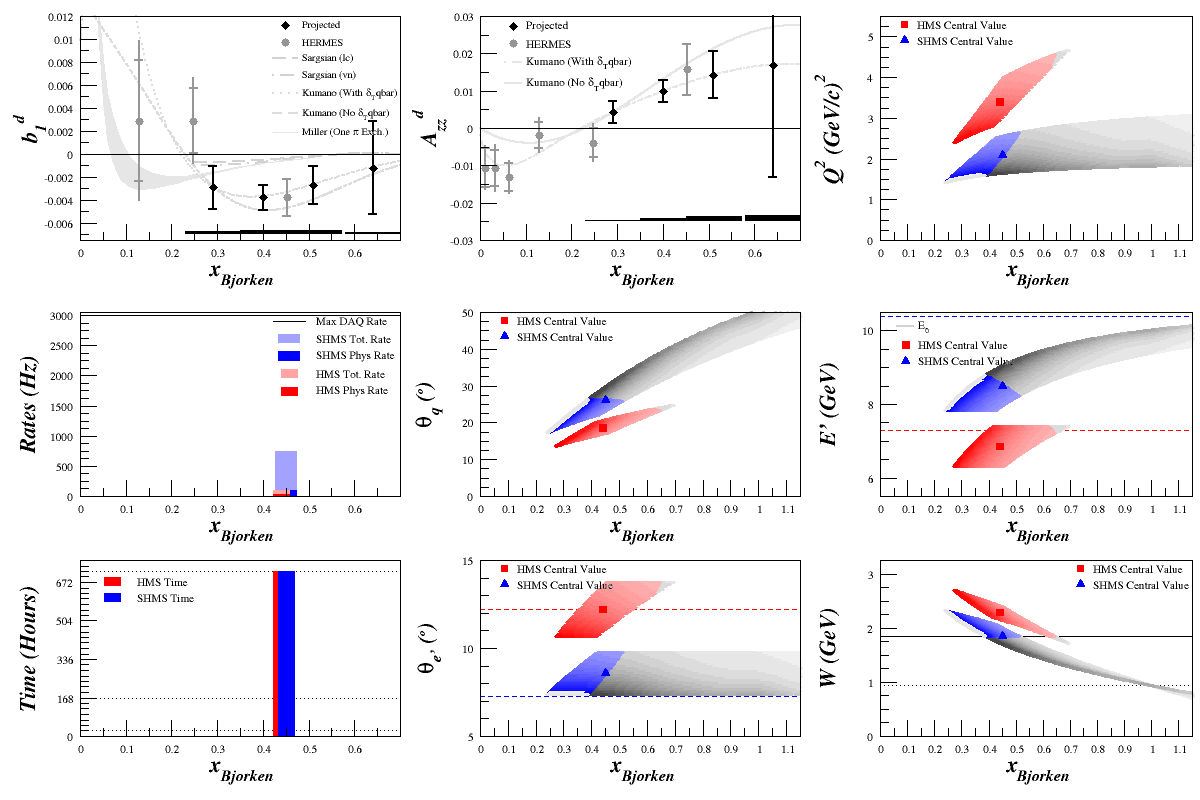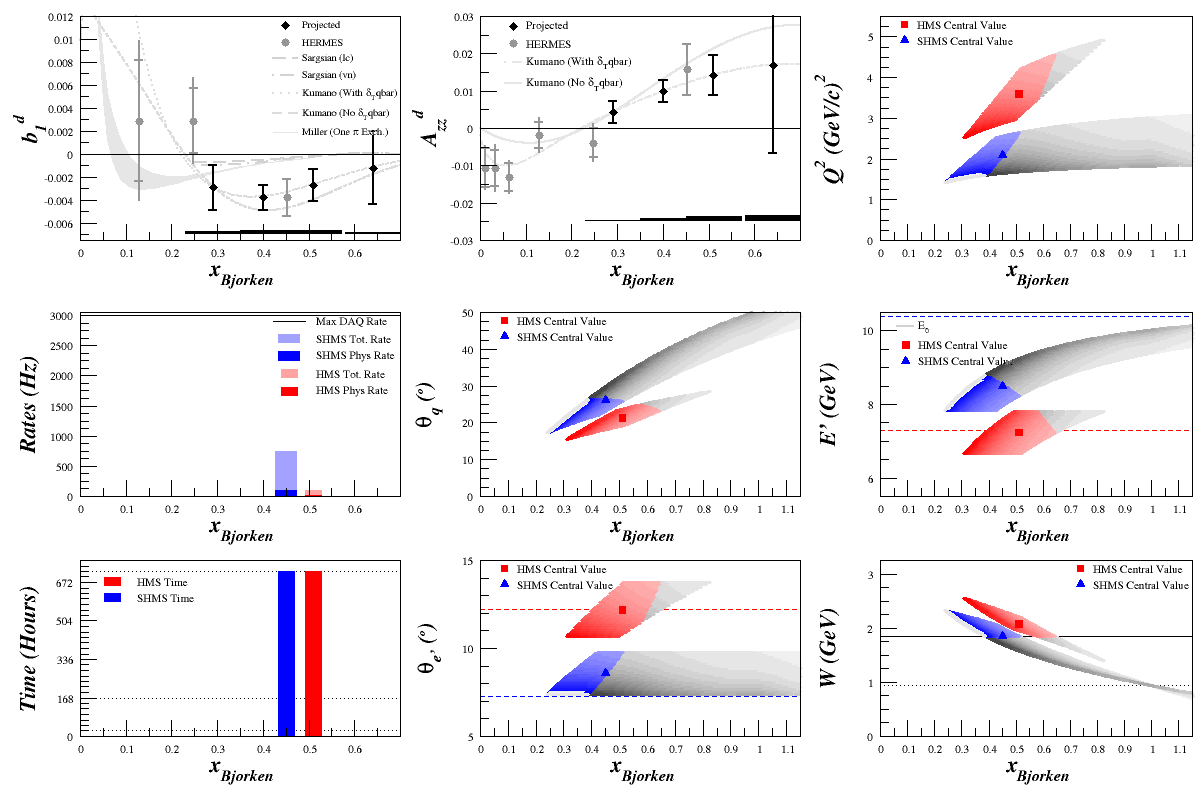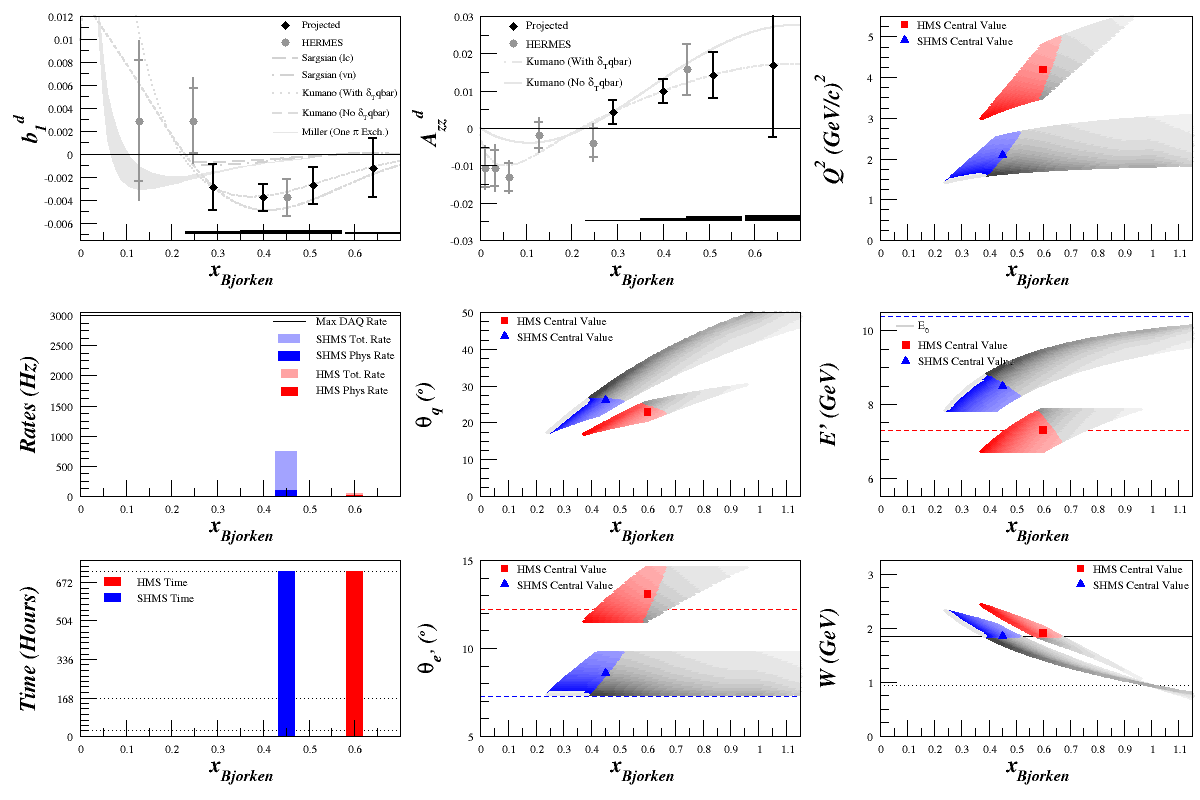
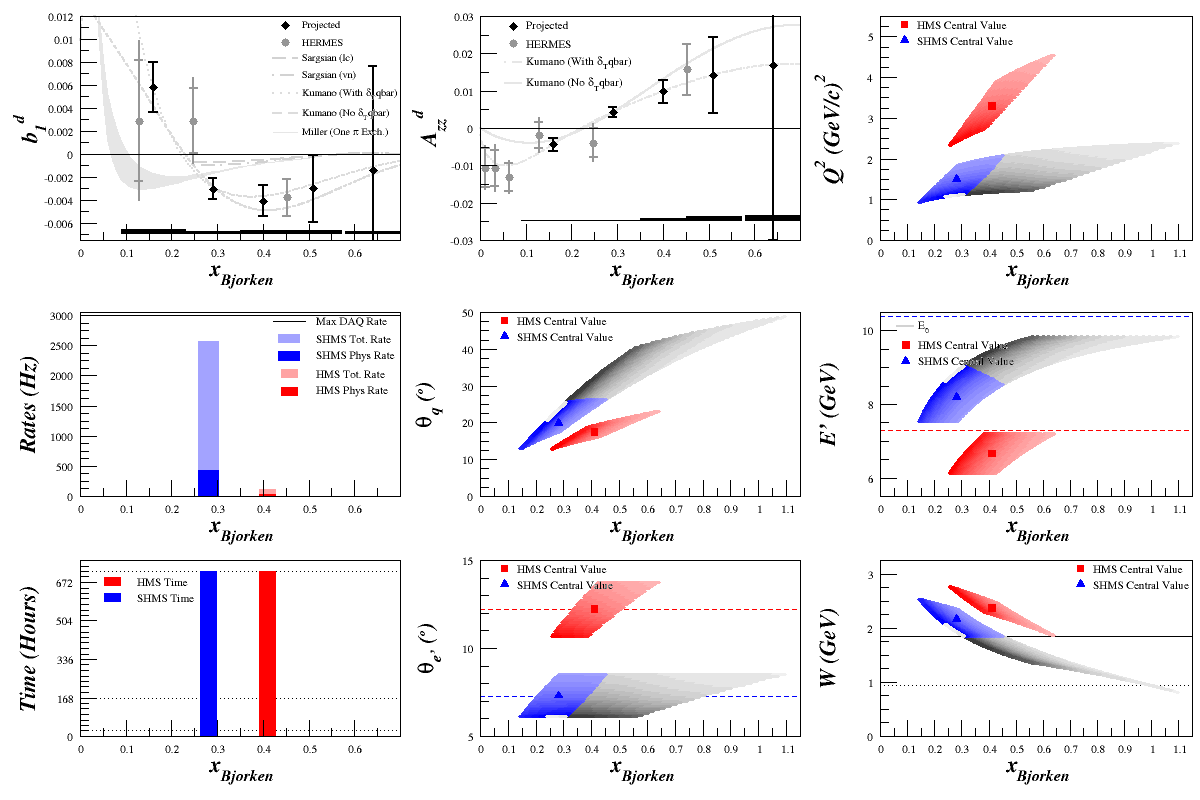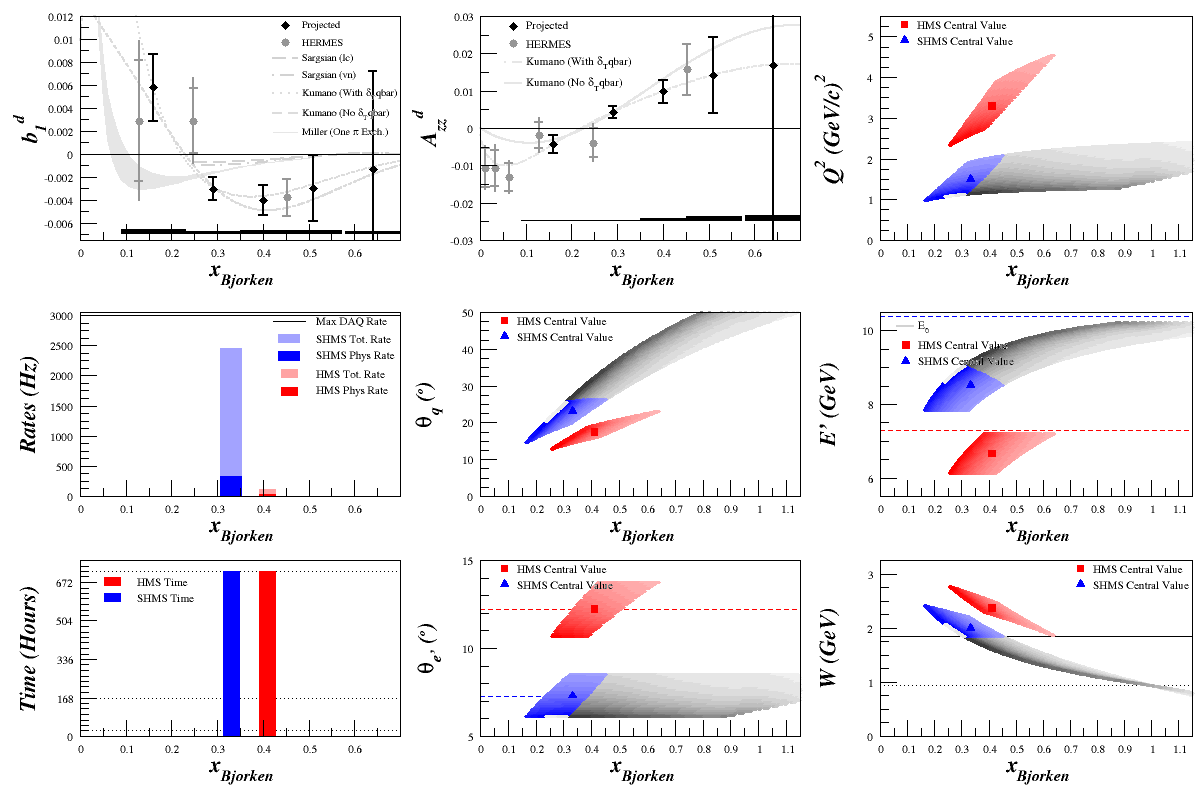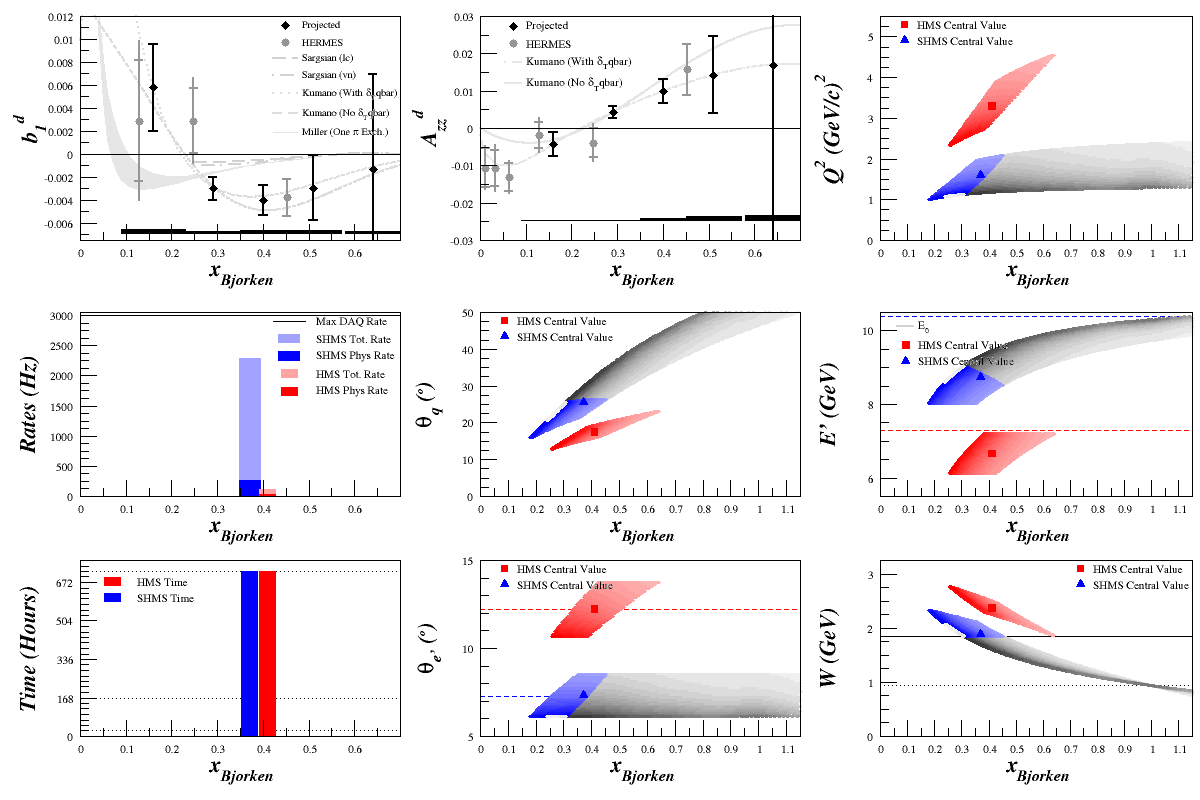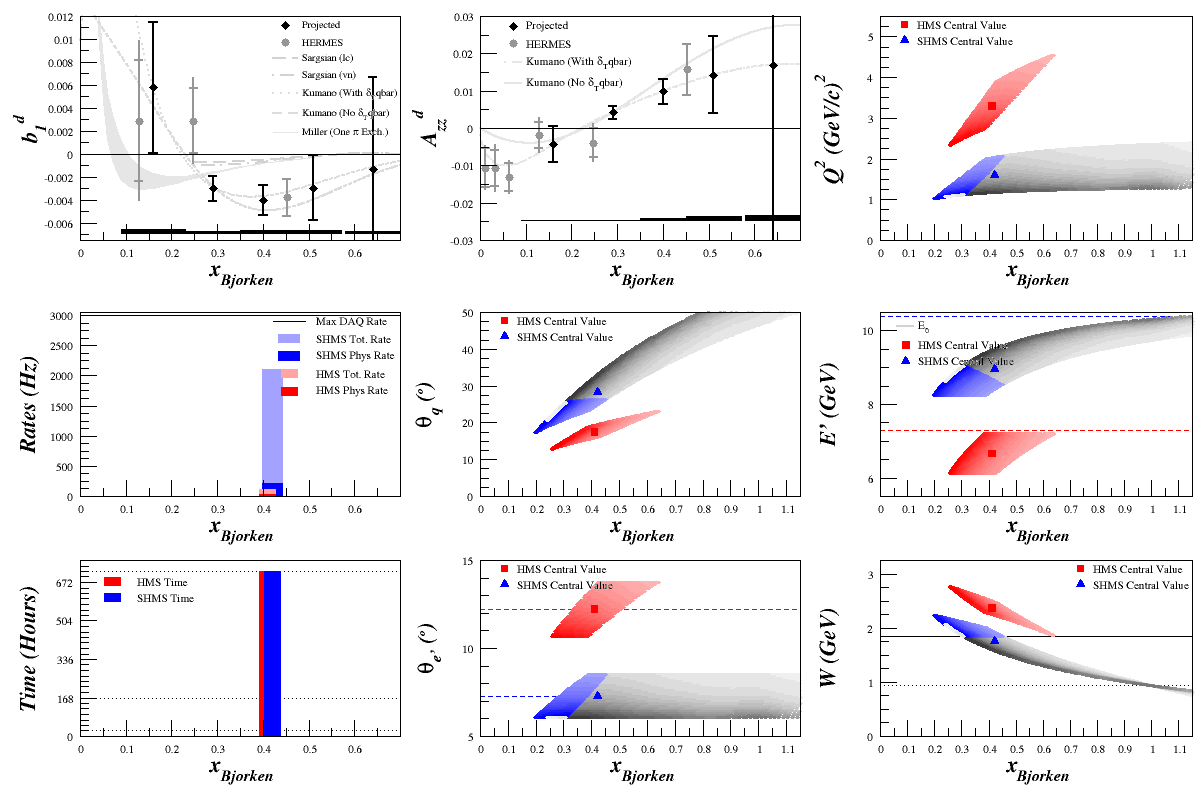
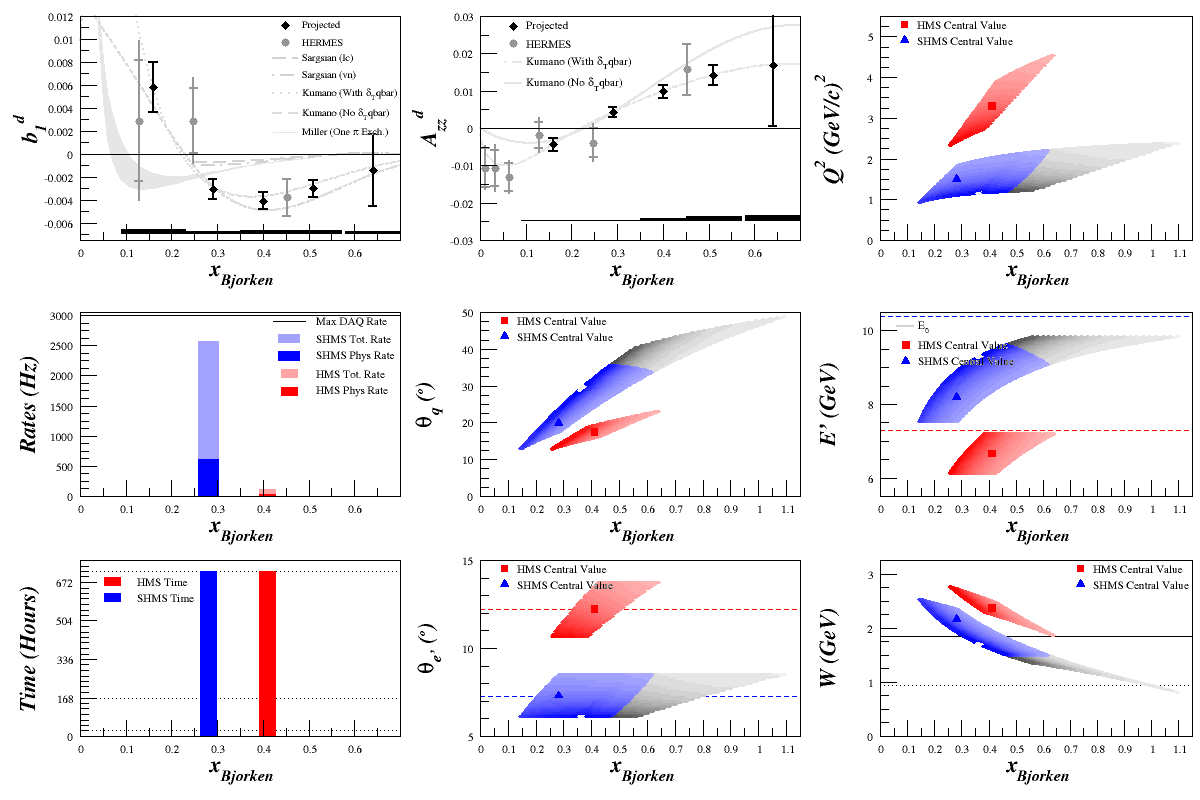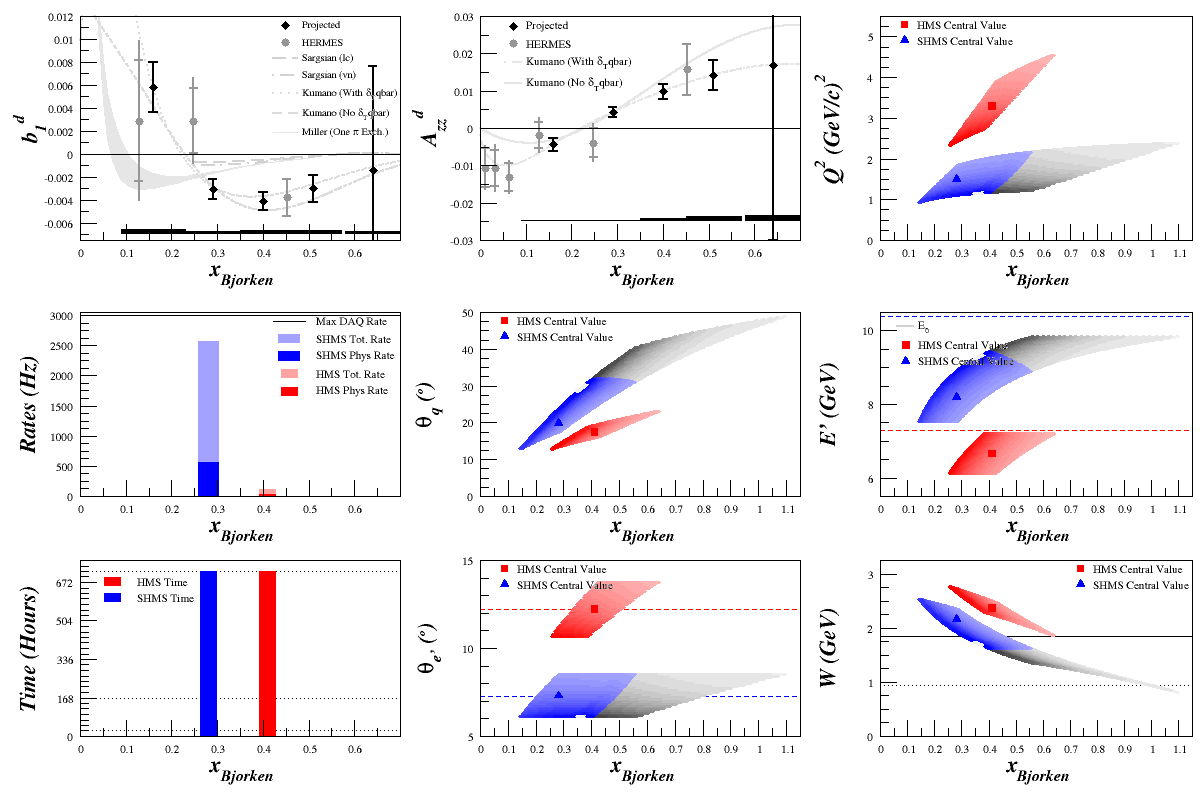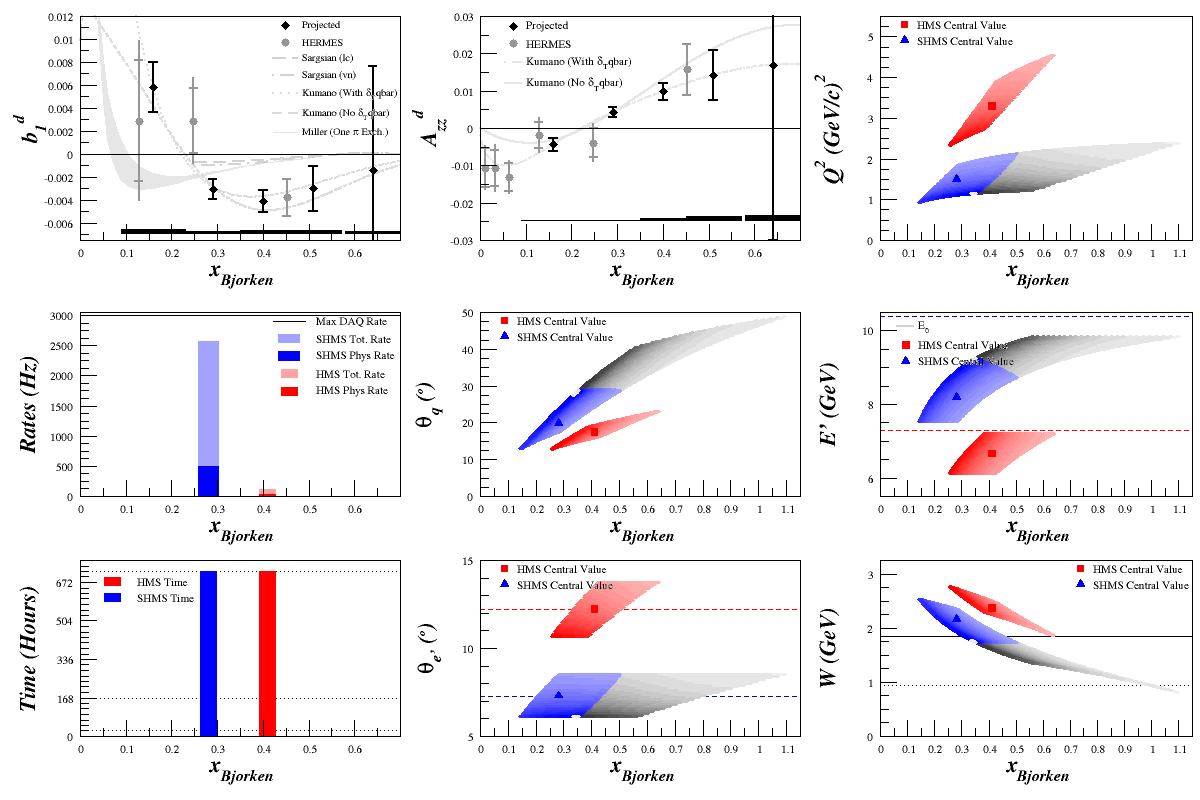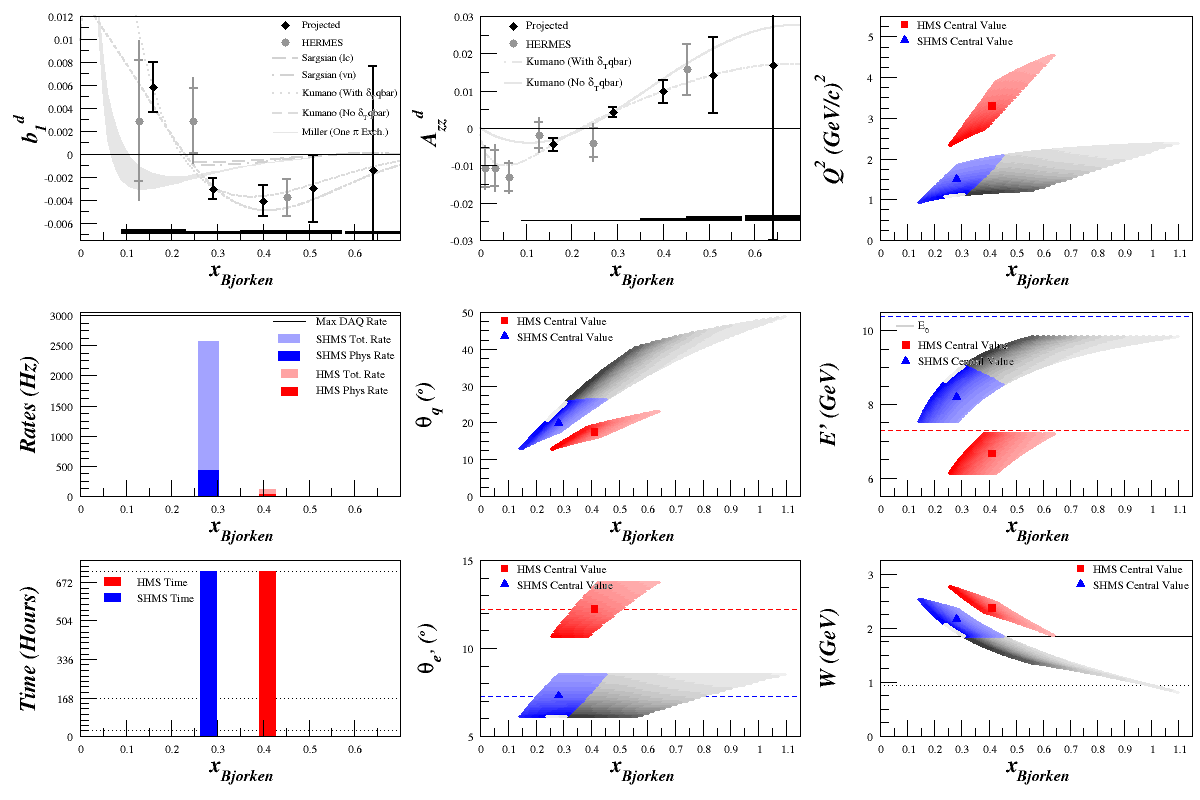
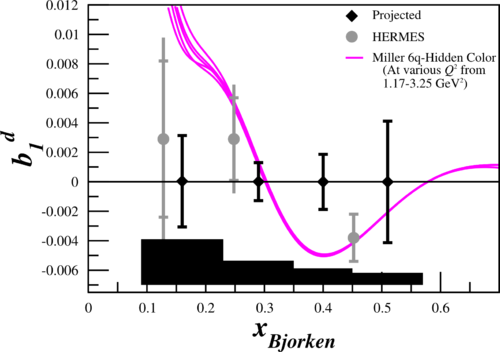
Fixing the systematic uncertainty by assuming that we'll have the same number of cycles for both spectrometers over the full length of time (36 cycles), if we don't lower the W cut and leave it at W>1.85 GeV, we get | Fixing the systematic uncertainty by assuming that we'll have the same number of cycles for both spectrometers over the full length of time (36 cycles), if we don't lower the W cut and leave it at W>1.85 GeV, we get | ||
| − | [[Image:2014-04-15-bestcase-w-185.png| | + | [[Image:2014-04-15-bestcase-w-185.png|500px]] |
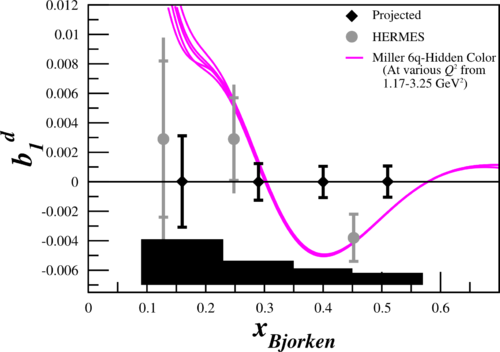
and if we can lower the W cut to W>1.5, we get | and if we can lower the W cut to W>1.5, we get | ||
| − | [[Image:2014-04-15-bestcase-w-150.png| | + | [[Image:2014-04-15-bestcase-w-150.png|500px]] |
Revision as of 11:13, 15 April 2014
Pzz=15% with ND3 Scenario
In a worst case scenario where we are stuck with Pzz=15% on an ND3 target, I looked into what single kinematics point we could spend all of our time on that would give us the best measurement. (Note: For each of these plots, the systematics were hard-wired to the specific kinematics we had listed for the proposal. Don't trust the systematics. The statistics are what's important.)
Individual frames for each of the plots below can be found here.
First I did a Q^2 scan of the SHMS
This was followed by an x scan of the HMS
and an x scan of the SHMS.
After each of these, it looked like our best point was to put the central SHMS at x=0.28 and Q^2=1.47 with the HMS at x=0.41 and Q^2=3.33. If we do that, and are ok lowering our W^2, we can increase the statistics in the x=0.4 point by opening up the cut
Results
Fixing the systematic uncertainty by assuming that we'll have the same number of cycles for both spectrometers over the full length of time (36 cycles), if we don't lower the W cut and leave it at W>1.85 GeV, we get
and if we can lower the W cut to W>1.5, we get