Difference between revisions of "Elong-14-05-09"
From HallCWiki
Jump to navigationJump to search (New page: =Recreating F&S Azz= Image:2014-05-09-fs-fig-7-3.png) |
|||
| Line 1: | Line 1: | ||
=Recreating F&S Azz= | =Recreating F&S Azz= | ||
| + | |||
| + | Using the Frankfurt and Strikman equation 7.3, | ||
| + | |||
| + | <math>A_{zz} = R(p_s) = \frac{3(k_t^2/2-k_z^2)}{k^2}\frac{u(k)w(k)\sqrt{2}+\frac{1}{2}w^2(k)}{u^2(k)+w^2(k)}</math> | ||
| + | |||
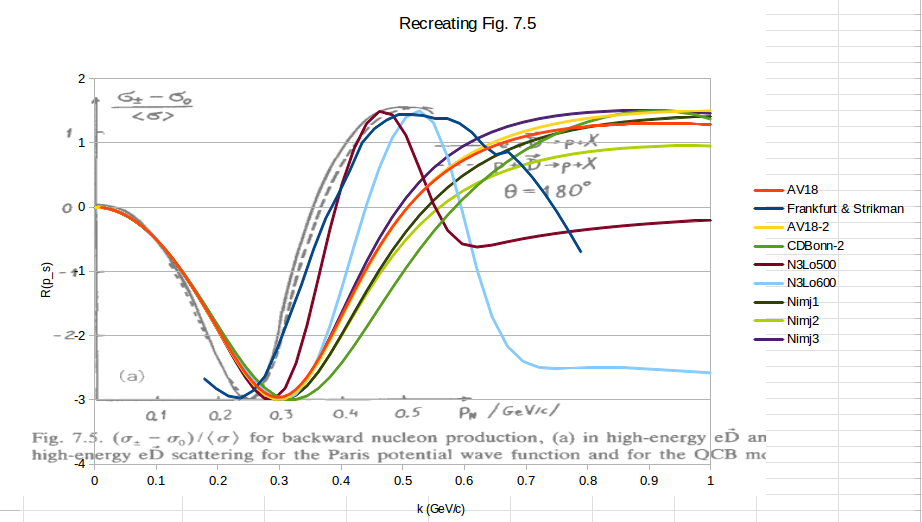
| + | I'm trying to recreated their Figure 7.5. I don't entirely understand what <math>k_t</math>, <math>k_z</math>, and <math>k</math> are, but if the ratio out front comes out to -3, I can recreate the D(e,e'p) at 180 degrees plot: | ||
[[Image:2014-05-09-fs-fig-7-3.png]] | [[Image:2014-05-09-fs-fig-7-3.png]] | ||
| + | |||
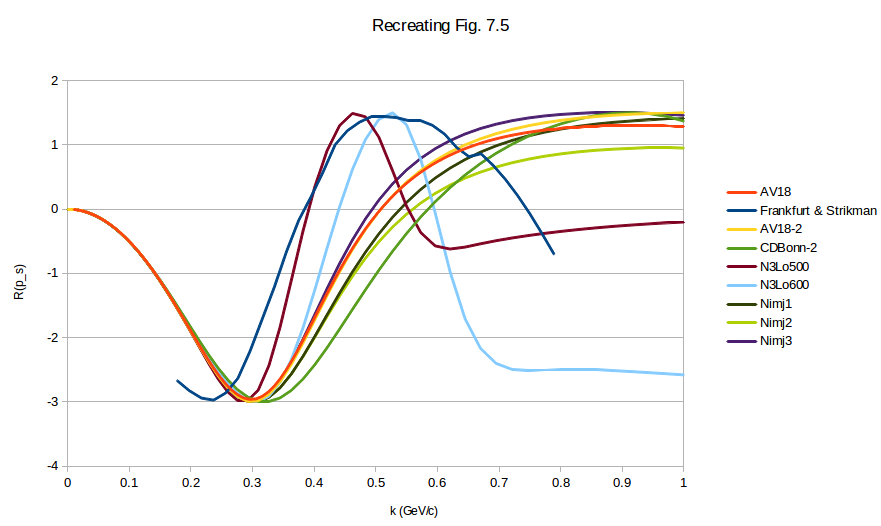
| + | Repeating this same process for all of the wavefunctions that I received from Donal, making sure that the low-k part of the D-state wavefunction is positive as is their convention in Figure 7.1, we see | ||
| + | |||
| + | [[Image:2014-05-12-wavefunctions-with-fs.png]] | ||
| + | |||
| + | [[Image:2014-05-12-wavefunctions-without-fs.png]] | ||
Revision as of 13:34, 12 May 2014
Recreating F&S Azz
Using the Frankfurt and Strikman equation 7.3,
<math>A_{zz} = R(p_s) = \frac{3(k_t^2/2-k_z^2)}{k^2}\frac{u(k)w(k)\sqrt{2}+\frac{1}{2}w^2(k)}{u^2(k)+w^2(k)}</math>
I'm trying to recreated their Figure 7.5. I don't entirely understand what <math>k_t</math>, <math>k_z</math>, and <math>k</math> are, but if the ratio out front comes out to -3, I can recreate the D(e,e'p) at 180 degrees plot:
Repeating this same process for all of the wavefunctions that I received from Donal, making sure that the low-k part of the D-state wavefunction is positive as is their convention in Figure 7.1, we see