Elong-14-05-15b

1981 Report

In Frankfurt and Strikman's 1981 report, they go into a bit more detail of how to make the cross section ratios. In particular, on page 258 there is an equation (3.19 and 3.20) for the ratio of cross sections with respect to k,

<math>r(\xi,k)=1+\left(\frac{3(k\xi)^2}{k^2}-1\right)\frac{u(k)w(k)\sqrt{2}+w^2(k)/2}{u^2(k)+w^2(k)}</math>

where <math>\xi</math> is the D-polarization in the D-rest frame, with <math>\xi=(1,\pm i, 0)/\sqrt{2}</math> for <math>\lambda_D=\pm 1</math>, and <math>\xi=(0,0, 1)</math> for <math>\lambda_D=0</math>. Assuming that <math>k\xi=\vec{k}\cdot\xi</math>, this leads to three possible ratios:

<math>r(\pm 1,k)=1+\left(\frac{3k_1^2-3k_2^2}{2\vec{k}\cdot\vec{k}}-1\right)\frac{u(k)w(k)\sqrt{2}+w^2(k)/2}{u^2(k)+w^2(k)}</math>

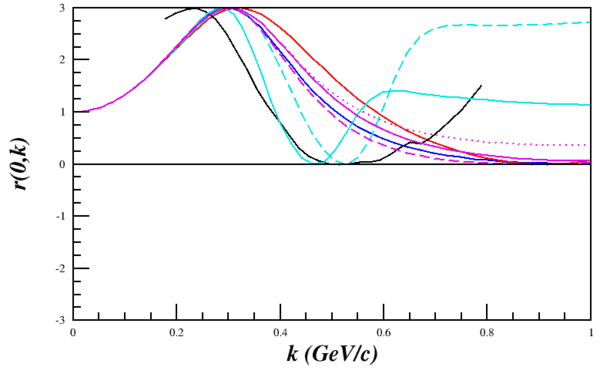
<math>r(0,k)=1+\left(\frac{3k_3^2}{\vec{k}\cdot\vec{k}}-1\right)\frac{u(k)w(k)\sqrt{2}+w^2(k)/2}{u^2(k)+w^2(k)}</math>.

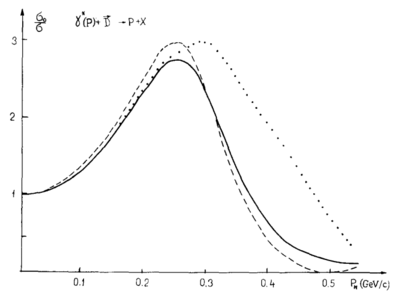
If I am understanding their kinematic variables correctly, then the virtual-photon points along the <math>\hat{z}</math> axis, so in the case of a nucleon being knocked-out at 180deg, <math>k_1=0, k2=0, k_3=|\vec{k}|</math>. This leads to, in the case of <math>r(0,k)</math> for their Fig. 3.9a,

<math>r(0,k)=1+2\frac{u(k)w(k)\sqrt{2}+w^2(k)/2}{u^2(k)+w^2(k)}</math>,

which can be recreated using the current wavefunctions.

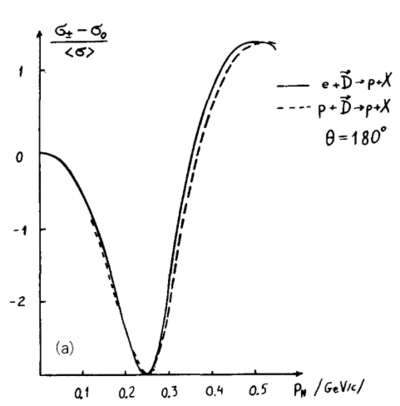
This would make their Fig. 7.5 from their 1988 report simply <math>A_{zz} = r(\pm 1,k)-r(0,k)</math> for D(e,e'p) with p at 180deg, which can also be reproduced.